 go back
go back
"Owls can rotate their head about their neck 270 degrees - they can see 360 degrees and more around them without ever moving their talons or feet."
So what? Humans can too. Try it.
The partitioning of time and space into 360 steps
The partitioning of a full circle as a representative of the whole into 360 degrees goes back to Babylonian times of farming and simple technology - 5000 years ago, around 3000 BC to 300 BC. Our current decimal number system is based on the number 10; their numerical system was based on the number 60.
In those days, the emphasis was not, as it is today, on arbitrarily precise mathematics, but on those principally so; it was not about calculations ( these were then neither possible nor needed ), but about division and construction of artifacts with available aids.
Within simple life, symmetry, dividing evenly, and fair and correct sharing, are of big, if not existential importance. Since reality itself is but an approximation on the mathematically correct, life did not depend on mathematically attainable precision or simplicity, but on the practically attainable. And the partitioning of a whole into 360 parts can be carried out relatively simply and mechanically, and it happens to be exceptionally practical mathematically as well.
The numbers 12, 60 or 360 can be divided evenly by almost any number that is useful in rural and simple municipal life, up to and including the 12 itself by 1, 2, 3, 4, 6, and 12, the higher ones in part by 5, 10, 15, 20, 30, and 60 as well; all together by a dozen ( again twelve ) factors, in which 60 represents 5 dozen and 360, for example, 30 dozen. The factors for 360 itself are numerous: 1, 2, 3, 4, 5, 6, 8, 9, 10, 12, 15, 18, 20, 24, 30, 36, 40, 45, 60, 72, 90, 120, 180, and 360.
The decimal or by-10-system, on the other hand, while easier for calculations, is not per se a system for measurement and allocation, but rather one for counting and computing; not only for systemic reasons, as with the later established dummy zero, but because people regularly have ten fingers. Therefore this system can be used very well to count, but not that well to share. It has, up to the number 10, only 4, and at that different, divisional factors as the by-twelve-system ( 1, 2, 5, 10 ); up to 100 there are only 9 altogether ( namely 1, 2, 4, 5, 10, 20, 25, 50, 100 ).
The number of divisional factors altogether is fundamentally higher in the open decimal system; however, these do not find practical application in simple life ( on the other hand, the number "three times ten" for example can be more easily understandable in some contexts than "three times twelve" - and can be very simply represented by showing both hands open three times ).
There is an even another divisional system, used until recently for everything that could be measured mechanically in daily life, which is to this day still in use here and there: the system of continued halving or bisection. With a beam balance, or a cord, it is very simple to halve quantities or lengths, then bisect these into quarters, eighths and sixteenths and so on: one quarter of a liter, a three quarter meter, one sixteenth of a inch, half a pound, and so forth.
The practicability of given calculating systems depends on the circumstances. This becomes interesting where different systems for counting and dividing overlap and conflict: the numbers of the by-12-system are easy to divide by three, this is difficult in the decimal or by-10-system, and in the halving or bisectional system. On the other hand, the decimal system copes well, for example, with the 5, the by-12-system far less; the halving system ditto.
In old times, however, for example in geometry - earth measurement - the expression in absolute correct numbers was not as important as that a given whole could be divided up, completely and evenly, with simple and available mechanical tools. And it actually is possible to divide a full circle into 2, 3, 4, 6, 8, 12 and 24 parts with nothing but a ruler and a pair of compasses ( note the 8! ). In fact, in Babylonian times, both could consist of one single tool, namely a string or cord with a peg on each end. A tense cord between two pegs serves as a ruler; if one then moves one peg, this describes a circle, in which the length of the cord determines the radius.
A full circle, representing a whole, can thus be drawn and divided into 6 parts with no more than a cord; this yields a naturally regular hexagon at the same time. By bisecting the angles ( just as simply, with a cord or pair of compasses ) a regular twelve-cornered dodecagon - or a dozen evenly spaced points on a circle - is attained. With that, the circle ( the whole ) is divided by 2, 3, 4, 6 and 12. The next bisection and division would yield 24; then 48, 96, 192, 384 - beyond the 24 however without much practical use.
A full circle, or a whole, can just as easily be divided into even parts of 2, 4, 8, 16, 32, 64, 128, 256 etc. by continued bisection, again with only cord or pair of compasses and ruler. This, too, becomes relatively pointless for all practical purposes after having arrived at 8 or 16 partitions - at the latest.
Only the factors 2 and 4 are common for both partitions; the 5 and 10, known from counting by fingers, are missing completely; and with them the 30, the 60, and finally the 360.
These factors, however, were necessary to represent time with this method, namely a year of about 360 days in 12 months to about 30 days each, as a cycle with 1 day / degree, at least to some accuracy ( and a very magical coincidence it is indeed - or is it? ).
For years, months and days could always have been observed via the natural cycles ( circular courses ) of celestial bodies; they were obviously spatial circles as well, which could be presented in numbers of 12, 30 and 360. Beyond this, there were further angles of technical importance besides 30°, such as 90° and 60°; these angles can also be presented by circular division. All in all, it centered around a favorable allocation of numbers, partitions, length and direction.
Here a first connection of circle and division and of time and space showed itself in the fields of astronomy, astrology and geometry ( ground survey ): the sky, a space, was moving over time; if man wanted to move in space and time, he needed a direction.
Temperature, for instance, though equally measured in degrees, is not circular.
In space, the orb was divided into four directions ( the north, the east, the south, the west ) then these were each bisected ( into northeast, southeast, southwest, northwest ), these again bisected when necessary ( into such as north by northeast ) and, after the invention of the magnetic compass, a still finer subdivision was carried out for navigational convenience - such as here into 120 graduation lines ( 30x4, 2x60 ).
The original mathematical and technical partitioning of the general spatial full circle into 60 subdivisions was expanded about 150 B. C. ( or even still earlier ) to 6x60 = 360 graduation lines ( degrees ) by astronomers which needed a higher resolution for more correct measurements, and this division ( with the still finer partitioning of the individual degree into 60 minutes = "small subdivision" and the individual minutes in turn into 60 seconds = "second subdivision" each ) was kept for the next two thousand years.
To arrive at a measure for time, on the other hand, one had to recognize the real division of the natural year ( the earth cycle ) into 12 months ( moon cycles ) with approximately 30 days ( sun cycles ) = 12x30 = 360 days each as an also possible 360-degree-division of a now temporal full circle ( cycle ).
For this division of a full circle or cycle into 360 degrees, needed for the partitioning of time and space, a circle could easily be divided into equally large sectors of exactly 6, then 12, and then 24 with a pair of compasses.
At first glance, 24 sectors are somewhat useless for the presentation of time; but one should note that pieces-of-eight and therefore regular octagons are thus possible for the first time, as they would be with a continual bisection of the same circle: the two systems of partitioning overlap here for the third time ( with 3x2 in 6, 3x4 in 12, 3x8 in 24 parts ).
Now, these 24 parts can be used for the division of time: in southern regions of the northern hemisphere the day, as smallest perceptible natural timecycle, is subdivided, the year over, relatively steadily and exactly by halves into brightness and darkness, day- and nighttime.
The idea of dividing the daily cycle into 2 equal sectors from sunrise till sundown and again from sundown to sunrise, and these again into 2 equal parts, before and after noon as a temporal mark - or midnight respectively - is not far fetched ( for the night, amongst other reasons, because night watchman is probably the second eldest profession of the world ). This way one has found the quartered circle again. As the practice showed, partitioning both day and night into a dozen time units each was suitable; together these yield 2x12 = 24 time units or hours per day.
These "hours" can, in turn, without difficulty, each be split into halves and quarters of an hour, or into even smaller units, all with help of a partitioned disc. To achieve this, one divides the circle up into 12 equal parts, mechanically, as discussed above; then divides each of these by free hand with four lines into 5 equal parts, to get the 5 and 10 as a factor; and so arrives at the 12x5 = 60 "minutes" of a full temporal circle ( in a spatial circle, on the other hand, a minute is represented by 6x60x60 = 21600 minutes to the whole ).
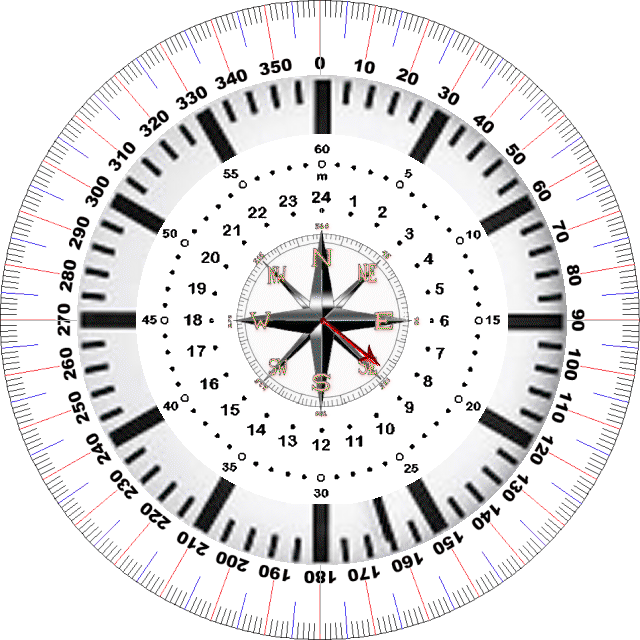
However, if the aim is to split the full circle into 360 sectors, for example for the temporal partitioning of the year or the spatial partitioning of the sky, bisecting the angles and segments beyond 24 does not achieve that goal, since 360/24 = 15 degrees is an odd number. Further bisection or 360/48 would have 7.5 degrees as a result.
15 however is 3x5; a segment of aspired 15 degrees can, with 2 lines, be divided into 3 parts, again by free hand, each of these again with 4 lines into 5 parts to arrive at 15.
[ By free hand respectively since, in a segment small enough, the practical execution by eye and hand becomes as correct as an elaborate construction; this is because, in a drawn construction, incorrigible inaccuracies very quickly add themselves up into large faults, often resulting in the complete uselessness of the outcome. On the other hand, with sufficient points, possibilities for adequately correct partitioning beyond the 24 can easily be arrived at empirically. See picture. For exercise, you can try costructing a 97° ( = 7° ) angle with a triangle with sides of 1, 1, and 1.5 radius ]
For better results, one can perform the small division on a second, larger concentric outer circle. If this is chosen big enough, the precision of the free division becomes larger than the tolerance of line width towards the center. In this way, 24 x15 lines yield 360 in a full circle altogether. The desired 360 graduation lines or divisions by degrees are thus obtained.
So, in principle, a full circle can have the following serviceable partitionings,
to be produced geometrically with sufficient precision:
This division of the time technically became important later on, when mechanical
watches were built with dials, and to this aim returned to the familiar
partitioning of the full circle; the same applies to the division of space
with the help of a magnetic compass. Of course, smaller or larger divisions,
as with the already, relatively arbitrarily, chosen time units of 1 hour
= ½ day / 12, could be employed as well ( and this is also
done ); but perhaps the temporal division of the hour into 60 minutes
- apart from the practical possibility of partitioning by the established
degree disc - holds some deeper reason.
Another natural cycle ( or rather rhythm ) can be found here: counting the rate of the adult heartbeat, during rest, from 1 to 60, there are about 60 heartbeats or "seconds" in a temporal minute ( and in that time, it will have pumped the complete volume of blood through the body approximately once ).
Therefore, 60x60 of these heartbeats yield about a twelfth of daylight time, two dozen of these a full daily cycle. The annual life rhythm of an adult person can therefore be represented almost completely in numbers of 12 ( 60/5, 360/30 ) or 24 ( 12x2 ) hours for 60x60 ( 12x5 x 12x5 ) heartbeats, 30 ( 60/2, 360/12 ) days of 24 hours each and 360 ( 6x60, 12x30 ) days per year, and this fairly correctly - and all done with the partitioning of a circle and a few whole numbers which can be derived from this mechanically.
There was - or seems to be - an obvious connection between predetermined natural human rhythms on one side and planetary times and courses on the other, describable in multiples and divisions of a numbers system based on the 12 or 60, which, if by chance or not, could also be shown as a natural partitioning by integers of a full circle or whole. This seemingly natural mathematical connection, between man and cosmos, was sufficiently exact to be deemed useful - with the necessary qualifications and corrections - for a few thousand years.
The simple division of a circle into 12, then 24, then 60 or 360 sectors can therefore represent at least the following phenomena, if sometimes only to first approximation:
Below the day, there is still the naturally predefined temporal subdivision
into daylight and night; this, however, already does not represent a cycle
in itself. Below that, the division of time - except maybe for the human
heartbeat - is more or less arbitrary, as perhaps is the division of space
with the two celestial axes standing vertically on each other near the equator,
which become literally pointless at the poles.
Nevertheless:
Understanding space as a circle and time as a cycle ( and so, in the end, the earth as a disc and time as eternity ), made, quite early in human history, one single instrument serviceable both for the division of time as for the dividing of space.
This way, it was possible to use the same instrument, the partitioned disc, to fix time and calculate it in hours, minutes and seconds, as well as to describe baselines - of artifacts for example - as circular, square, hexagon or octagon, and to fix and represent directions for navigating.
And, among other things, it seems a possible explanation why astrology was developed with twelve signs of the zodiac.
The question remains, whether life, especially human life, would have developed the way it has, if the mass, the distance and momentum of the heavenly bodies involved, in the period of time in question, had not by chance generated such harmonious, self-dividing periods.
"The World is Sound."
Fun fact:
Human 360 degrees round vision:
Plant your feet a foot apart, then turn your head to the right as far as you can, then as far as possible to the left. You should be able to look straight out backwards both ways: that's 360 degrees.
You will notice that your vision now covers more than 360 degrees - in fact, the right and left vision overlap by about 90 degrees.
That's 450 degrees for you.